Entangled polymers
Entangled polymers
 Solutions and melts of polymers (long macromolecules of diverse topology formed by repeating molecular units) are prime ingredients in modern materials, in part because of the spectacular range of controllable viscoelastic behaviors they exhibit. Unfortunately, achieving a first-principles theoretical understanding of the the viscoelasticity and microscopic dynamics of strongly entangled synthetic and biological polymer liquids is an extremely challenging problem — at a coarse-grained level the task is to describe a dense liquid of diffusing, interpenetrating random walks. For highly extended objects topological constraints, the interplay between the long-range connectivity of the constituent parts and their inability to pass through each other, dominates viscoelasticity and relaxation, but capturing these topological interactions within a microscopic framework has generally been impossible.
Solutions and melts of polymers (long macromolecules of diverse topology formed by repeating molecular units) are prime ingredients in modern materials, in part because of the spectacular range of controllable viscoelastic behaviors they exhibit. Unfortunately, achieving a first-principles theoretical understanding of the the viscoelasticity and microscopic dynamics of strongly entangled synthetic and biological polymer liquids is an extremely challenging problem — at a coarse-grained level the task is to describe a dense liquid of diffusing, interpenetrating random walks. For highly extended objects topological constraints, the interplay between the long-range connectivity of the constituent parts and their inability to pass through each other, dominates viscoelasticity and relaxation, but capturing these topological interactions within a microscopic framework has generally been impossible.
 In polymer physics the most popular and well-developed approaches for linear macromolecules (rigid rods and flexible chains) are built on the phenomenological ideas of deGennes, Doi, and Edwards. There a single-polymer dynamic mean-field theory is constructed in which inter-chain interactions are replaced by ansatz with an in- finitely strong confining “tube” that strictly forbids large-scale poly 2displacement transverse to the polymer backbone. Multiple corrections to the quiescent tube model which enhance polymer mobility and reduce motional anisotropy are experimentally important, but are not derived in a rigorous way. Both the corrections and even the tube itself rely on inspired guesswork to encode the consequences of topological constraints on the physical motion of a single tagged polymer.
In polymer physics the most popular and well-developed approaches for linear macromolecules (rigid rods and flexible chains) are built on the phenomenological ideas of deGennes, Doi, and Edwards. There a single-polymer dynamic mean-field theory is constructed in which inter-chain interactions are replaced by ansatz with an in- finitely strong confining “tube” that strictly forbids large-scale poly 2displacement transverse to the polymer backbone. Multiple corrections to the quiescent tube model which enhance polymer mobility and reduce motional anisotropy are experimentally important, but are not derived in a rigorous way. Both the corrections and even the tube itself rely on inspired guesswork to encode the consequences of topological constraints on the physical motion of a single tagged polymer.
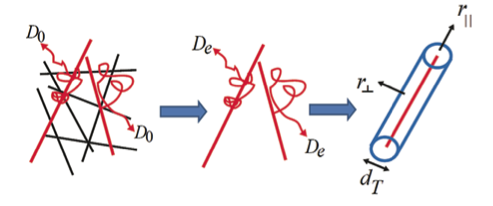
 Starting from a microscopic theory for the dynamics of dense solutions of infinitely thin rods worked on by Szamel in the early 90’s, we developed a theory for the dynamics and tube confinement of entangled rigid rods and flexible linear chains. By combining this theory with ideas from glassy physics for describing non-gaussian fluctuations we predicted an anharmonic form for the confining tube in excellent agreement with simulated chains and experiments on semiflexible biopolymers.
Starting from a microscopic theory for the dynamics of dense solutions of infinitely thin rods worked on by Szamel in the early 90’s, we developed a theory for the dynamics and tube confinement of entangled rigid rods and flexible linear chains. By combining this theory with ideas from glassy physics for describing non-gaussian fluctuations we predicted an anharmonic form for the confining tube in excellent agreement with simulated chains and experiments on semiflexible biopolymers.
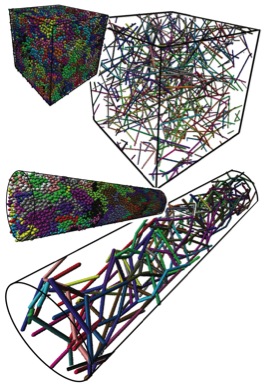
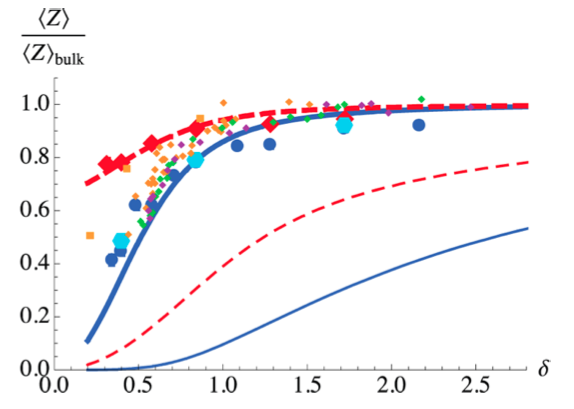 In 2013 we generalized our theory to make quantitative predictions for the dependence of the tube diameter on anisotropic orientational distributions of the primitive path steps. We also showed that the coarse grained primitive path segments can be analyzed under a Green-Kubo formalism to explore how intermolecular uncrossability constraints contribute to entanglement elasticity.
In 2013 we generalized our theory to make quantitative predictions for the dependence of the tube diameter on anisotropic orientational distributions of the primitive path steps. We also showed that the coarse grained primitive path segments can be analyzed under a Green-Kubo formalism to explore how intermolecular uncrossability constraints contribute to entanglement elasticity.
This generalization led to a collaboration with with Rob Riggleman and Karen Winey, in which we worked to understand how geometrical confinement (in thin film or cylindrical geometries) affects the polymer entanglement network.